The answer is simple if you understand that there is a number y always between 3 and x, but NEVER equal to either x > y > 3 In the Real or Rational numbers, no matter how close x is to 3, there is a way to slip a y "in between x and 3θ = Angle between two radii R = Radius of outer circle r = Radius of inner circle θ = Angle between two radii R = Radius of outer circle r = Radius of inner circle 6 Statistics Formulas Mean am = a1 a2a3 a4 4 = n ∑ 0a n a m = a 1 a 2 a 3 a 4 4 = ∑ 0 n a nQuestion The equation x3 3xy y3 = 1 is solved in integers Find the possible values of xy Found 3 solutions by Alan3354, Edwin McCravy, richard1234

X3 9y3 3xy X Y এর উৎপ দক ব শ ল ষণ কর Brainly In
X^3+y^3+z^3=(x+y)^3-3xy(x+y)+z^3
X^3+y^3+z^3=(x+y)^3-3xy(x+y)+z^3-Let's Summarize The minilesson targeted in the fascinating concept of the cube of a binomial The math journey around the cube of binomial starts with what a student already knows, and goes on to creatively crafting a fresh concept in the young mindsNCERT Solutions Class 9 Maths Chapter 2 – Polynomials Exercise 25 are given here These NCERT Maths solutions are created by our subject experts which makes it easy for students to learn The students use it for reference while solving the exercise problems The fifth exercise in Polynomials Exercise 25 discusses the Algebraic Identities
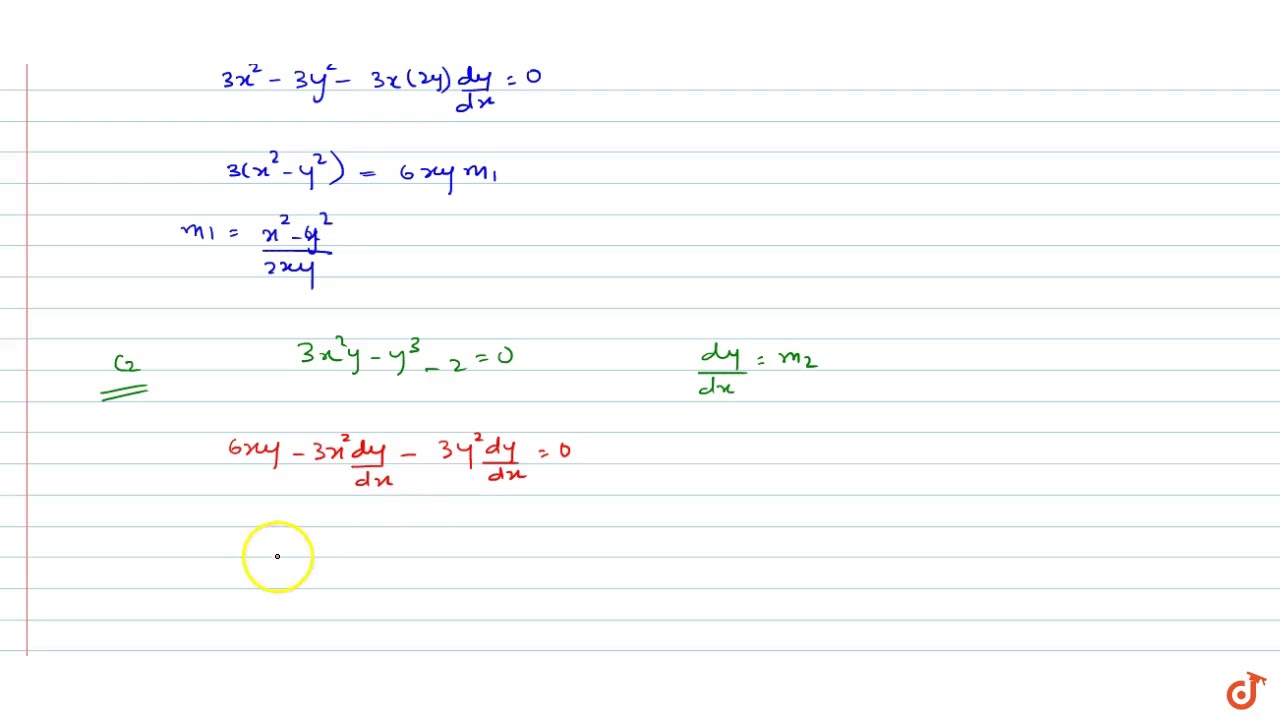



The Two Curves X 3 3xy 2 2 0 And 3x 2y Y 3 2 0 Youtube
X a /x b = x ab = 1/x ba;The formula is (xy)³=x³y³3xy(xy) Proof for this formula step by step =(xy)³ =(xy)(xy)(xy) ={(xy)(xy)}(xy) =(x²xyxyy²)(xy) =(xy)(x²y²2xy find x^3y^3 The coordinates of point Y are giving The midpoint XY is (3,5) Find the coordinates of point X
Polynomial Examples Find the remainder when x 4 x 3 – 2x 2 x 1 is divided by x – 1 Solution Here, p(x) = x 4 x 3 – 2x 2 x Give possible expressions for the length and breadth of each of the following rectangles, in which their areas are given (i) Area 25a2 – 35a 12 (ii) Area 35y2 13y – 12 Solution (i) We have, area of rectangle = 25a 2 – 35a12 = 25a 2 – a – 15a12 • (x – y) 3 = x 3 – y 3 – 3xy(x – y) • x 3 y 3 z 3 – 3xyz = (x y z)(x 2 y 2 z 2 – xy – yz – zx) Also, check the NCERT Solutions for Class 9 Maths Chapter 2 from the
1 Explanation We know that algebraic formula, (x y) 3 = x 3 y 3 3xy (x y) put the value of x y in given equation given, x y = 1 1 = x 3 y 3 3xy X 1?2 View Full Answer Here, exponent of every variable is a whole number, but x 10 y 3 t 50 is a polynomial in x, y and t, ie, in three variables So, it is not a polynomial in one variable Ex 21 Class 9 Maths Question 2




If X Y 1 Then Find The Value Of X 3 Y 3 3xy Maths Continuity And Differentiability Meritnation Com




Factorise X3 9y3 3xy X Y Brainly In
If x y z = 0, show that x3 y3 z3 = 3xyz Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to getX 3 y 3 3xy = 1CBSE NCERT Notes Class 9 Maths Polynomials Show Topics Class 9 Maths Polynomials Algebraic Identities Algebraic Identities Algebraic identity is an algebraic equation that is true for all values of the variables occurring in it ( x y) 2 = x2 2 xy y2 ( x – y) 2 = x2 – 2 xy y2 x2 – y2 = ( x y) ( x – y)



1




X Y 3 X3 Y3 3xy X Y Proof It Maths Polynomials Meritnation Com
We know that algebraic formula, (x y) 3 = x 3 y 3 3xy (x y) put the value of x y in given equation given, x y = 1 1 = x 3 y 3 3xy X 1 ⇒ x 3 y 3 3xy = 1 Previous Question Next Question Your comments will be displayed only after You are very important to us For any content/service related issues please contact on this number / Mon to Sat 10 AM to 7 PM Answer (xy)^3 = (xy)^2 (xy) = (x^2y^22xy) (xy) = x^3xy^22x^2yx^2yy^32xy^2 = x^3y^33x^2




If X Y 7 And X 3 Y 3 133 Find X 2 Y 2 Brainly In




Verify X Y 3 X3 Y3 3xy X Y Brainly In
State reasons for your answer Ans (i) 4x 2 – 3x 7 ⇒ 4x2 – 3x 7x° ∵ All the exponents of x are whole numbers ∴ 4x 2 – 3x 7 is a polynomial in one variable (ii) ∵ All the exponents of yXy yz zx is a quadratic polynomial in x, y and z If x y = 12, and xy = 27, then find the value of x3 y3 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries
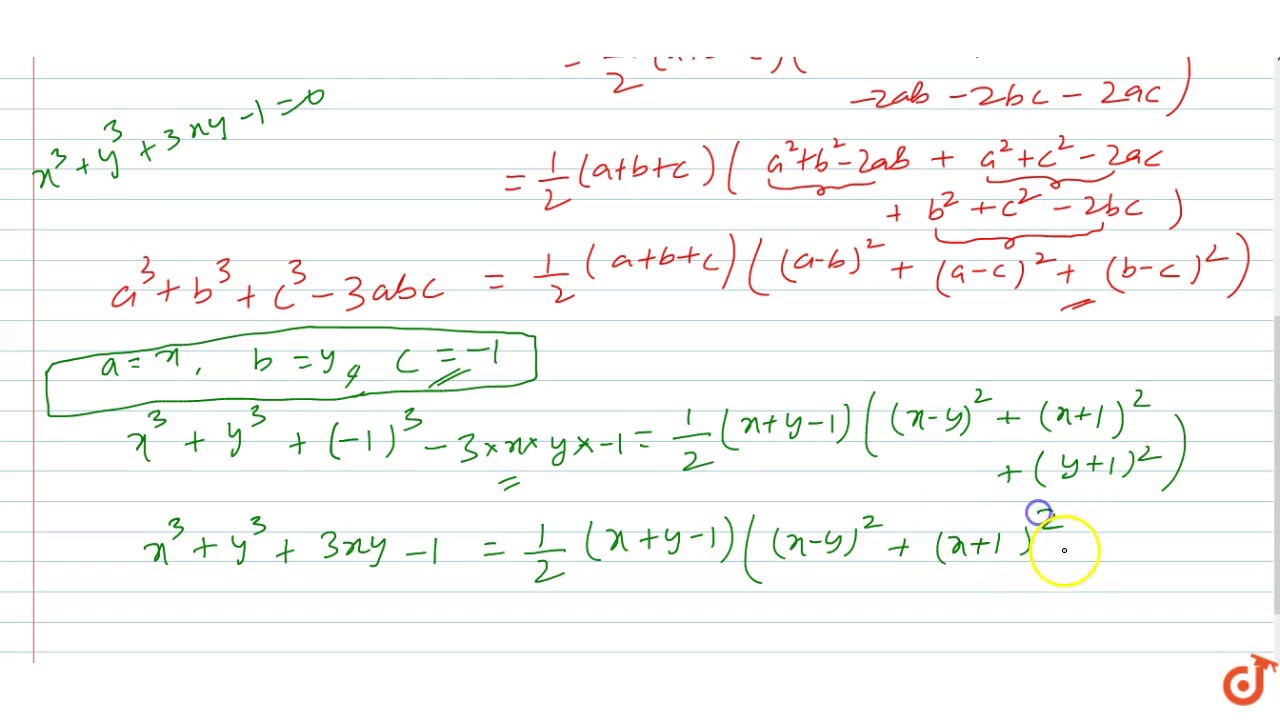



Q 16 Locus All Point P X Y Satisfying X 3 Y 3 3xy 1 Consists Of Union Of A A Youtube




If Math 3x 4y Sqrt Xy Math How Can You Prove That Math X 2 Y 2 Xy Math Quora
Login Create Account (xy) 3 = x 3y 3 3xy(xy)(4) 3 =x 3y 3 3(21)464=x3y3252x 3y 3 =x 3y 3 =1 0Get FREE NCERT Solutions for Class 9 Maths Chapter 2 Polynomials Ex 25 We have created Step by Step solutions for Class 9 maths to help you to revise (x y) 3 = x 3 3x 2 y 3xy 2 y 3 = x 3 y 3 3xy(x y) So 9 3 = x 3 y 3 3*10*9 x 3 y 3 = 729 270 = 459 Alan



Find The Critical Points Of F X Y X 3 Y 3 3xy Chegg Com




Second Partials Test For F X Y X 3 3xy Y 3 Youtube
Ĺet x^yy^x=(xy)^3=x^3y^33xy(xy) Or,x^yy^x=x^3y^39xy again x=3y so x^3y^39xy=(3y)^3y^39(3y)y=2727y9y^2y^3y^39y^227=5427y Now we got 54 27y=27, or 27y=27,y=1 Then x=31=2 The values of x& y only satisfy xy=3 and doen't satisfy x^yy^x=27 So there is no solution for the values of x & yX y is a binomial in which x and y are two terms In mathematics, the cube of sum of two terms is expressed as the cube of binomial x y It is read as x plus y whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ( x y) 3 = x 3 y 3 3 x 2 y 3 x y 21 Which of the following expressions are polynomials in one variable and which are not?



How To Solve Math Xy 2 Dy X 3 Y 3 Dx 0 Math Quora




X3 9y3 3xy X Y এর উৎপ দক ব শ ল ষণ কর Brainly In
this formula can be derived from (x y) 3 = x 3 y 3 3xy (x y) x 3 y 3 = (x y) 3 3xy (x y) x 3 y 3 = (x y) (x y) 2 3xy = (x y) x 2 y 2 2xy 3xy = (x y) (x 2 xy y 2 ) Was this answer helpful?We know that (x y) 3 = x 3 y 3 3xy(x y) Using Identity VII ⇒ x 3 y 3 = (x y) 3 3xy(x y) x 3 y 3 = (x y){(x y) 2 3xy} ⇒ x 3 y 3 = (x 5 Linear Polynomial A polynomial of degree one is called a linear polynomial eg, x √7 is a linear polynomial in x, y and z √2 µ 3 is a linear polynomial in µ 6 Quadratic Polynomial A polynomial of degree two is called a quadratic polynomial eg;



How To Solve The General Solution Of X 3 Y 3 Dx Xy 2dy 0 Quora




How To Evaluate Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube
Here given are, XY=7, let's say it is formula 1 in order to make it kinda convenient and easy to understand And XY=10, let's mark it as 2 Now, see formula 1 can be also written as,Telangana SCERT Class 9 Math Chapter 2 Polynomials and Factorisation Exercise 25 Math Problems and Solution Here in this Post Telanagana SCERT Class 9 Math Solution Chapter 2 Polynomials and Factorisation Exercise 25Maths Tutor Chandigarh, Chandigarh, India 486 likes 7 talking about this My self Sujit Adhikari from Chandigarh If you need Mathematics teacher, please feel free to contact me at
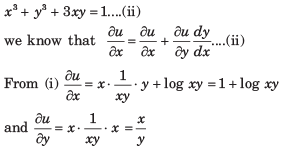



If U X Log Xy Wherex3 Y3 3xy 1 Then Du Dx Is Equal Toa B C D Correct Answer Is Option A Can You Explain This Answer Edurev Electronics And Communication Engineering Ece



If X 3 Y 3 9 And X Y 3 Then What Is The Value Of X 4 Y 4 Quora
Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeT 3 = 91 3 x y (x y) = 91 3 x y t Now from result 1 we know xy in terms of t Substituting in above, we get cubic in t, t 3 − 75 t 1 = 0 On solving for t, we get 3 solns t=7,, Now we know t = xy, on substituting for t in result 1 ,we get the value of xy As we know now xy and (xy) we can solveXXX=15 is equivalent to 3x=15 If you divide each side of the equation by 3, you get x=5 Then, since you know the value of x, you can combine like terms in xyy=35 xyy=35 would be equivalent to 2yx=35 You can then substitute 5 for x, making the equation 2y5=35 Subtract 5 from each side to get 2y=30




If X Y 7 And X3 Y3 133 Find I Xyii X Y Brainly In
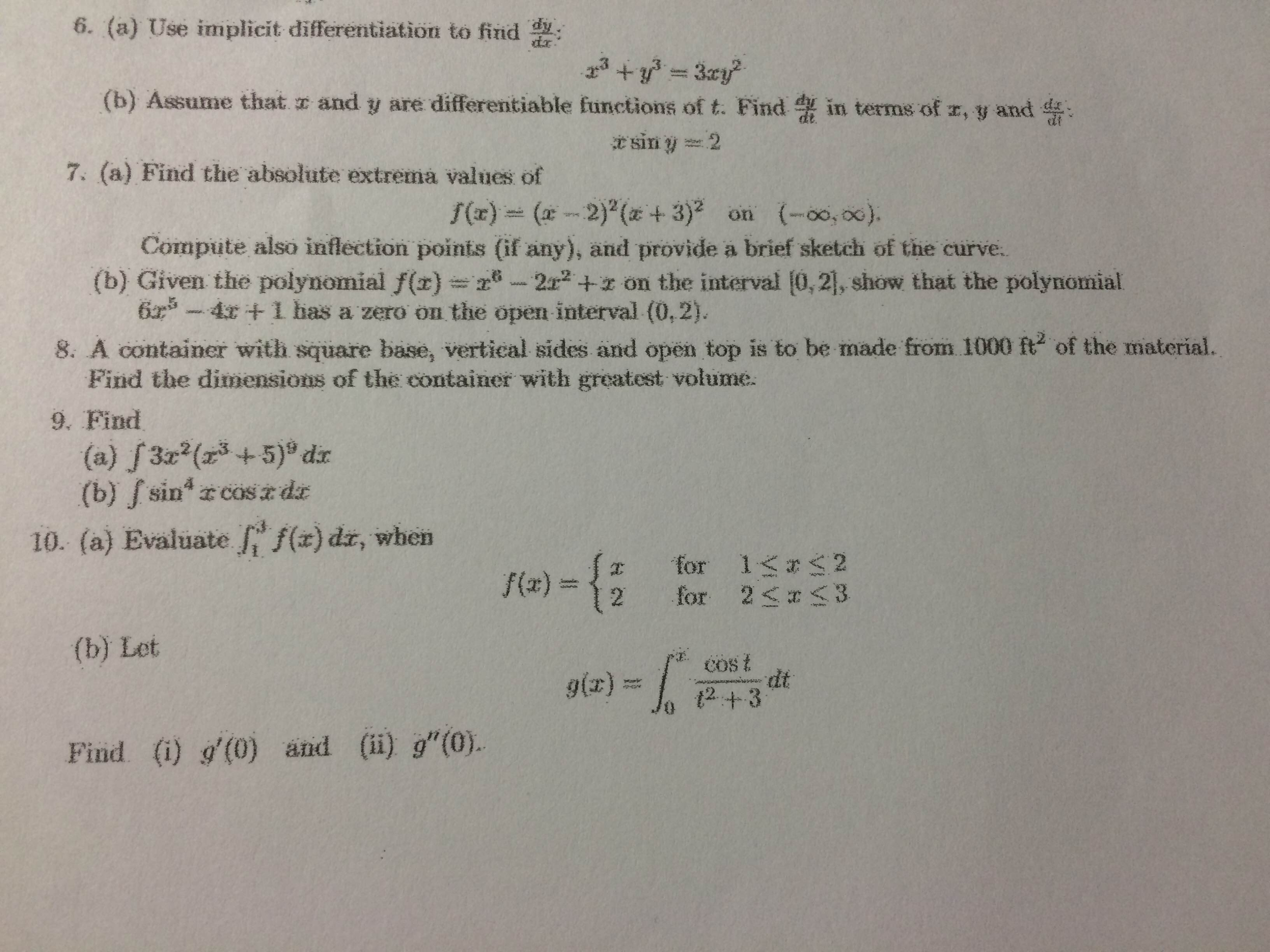



6 A Use Implicit Differentiation To Find Dy Dx Chegg Com
Quadratic equation, for any given x if ax 2 bx c =0 then x has 2 solutions x=(b√(b 2 4ac)/2a, x=(b√(b 2 4ac)/2a x a y b is not equal to (xy) abPolynomial equation is of four types Monomial This type of polynomial contains only one term For example, x 2 , x, y, 3y, 4z Binomial This type of polynomial contains two terms For example, x 2 – 10x Trinomial This type of polynomial contains three terms For example, x 2 – 10x9 Quadratic Polynomial This type of polynomialX 0 =1 x a y a = (xy) a, 2 2 3 2 = 6 2;



If X 3 Y 3 9 And X Y 3 Then The Value Of X 4 Y 4 Please Answer Honestly Don T Watch Others Quora




Expand X X Y 3 3xy X Y
Using formula, (x – y) 3 = x 3 – y 3 – 3xy(x – y) (99) 3 = (100 – 1) 3 = (100) 3 – 1 3 – (3 × 100 × 1) (100 – 1) = – 1 – 300(100 – 1Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange If xy = 4 and xy = 21 then find the value of x3 y3 Maths Polynomials NCERT Solutions;




What Are The Factors Of X Y 3 X3 Y3 Brainly In



Identify The Critical Points For The Function F X Y 3xy X 3 3y 2 Socratic
(xy) 3 = x 3 y 3 3xy(x y) x 2 y 2 = (x y)(x y) x2 = 1/x 2, 24 = 1/16 = 1/2 4 (x a)(x b) = x ab; (ii) (2a – 3b) 3 = (2a) 3 – (3b) 33(2a)(3b)(2a3b) Using identity (xy) 3 =x 3y 33xy(xy) = 8a 327b 318ab(2a3b) = 8a 3 – 27 b 3 – 36a 2 b 54ab 2 =8a 3 – 36a 2 b 54ab 2 – 27 b 3 Question 7 Evaluate the following using suitable identities (i) (99) 3Start your 48hour free trial to unlock this answer and thousands more Enjoy eNotes adfree and cancel anytime



Simplify The Expression X 3 Y 3 X Y 2 3xy X Y 2 3xy X 3 Y 3 X Xy X 2 Y 2 Sarthaks Econnect Largest Online Education Community




2 2 Y 3 Cheap Online
(x y z) 2 = x 2 y 2 z 2 2xy 2yz 2zx (x y) 3 = x 3 y 3 3xy(x y) (x – y) 3 = x 3 – y 3 – 3xy(x – y) x 3 y 3 z 3 – 3xyz = (x y z)(x 2 y 2 z 2 – xy – yz – zx;Now your easily get the answer X×3 Y×3 = 9×3 5×3 this implies, 27–15=12 Hence 12 is the right answer for this I would suggest a trick for that make a pair in which XY =4 and choose that no if we multiply both then they give less than 45 After 2–3 attempt if you are beginners you can easily find the correct pair for all equationsAnswer(xy)³=x³y³3xy(xy) (xy)³= (xy)(xy)(xy) (xy)²(xy) = (x²y²2xy) (xy) = x(x²y²2xy) y(x²y²2xy) = x³xy²2x²yx²yy³2xy²




Verify X3 Y3 And X3 Y3 Std 9 Maths Ex 2 5 Q 9 Youtube




X Y 3 X 3 Y 3 3xy X Y Verify Maths Meritnation Com
Near (x, y)=(3245, 197), (309, 845), (25, 9) and (2365, 15) And since the two equations can be combined into a quartic in x, (or a quartic in y) and a quartic has 4 roots in the complex numbers and we have found 4 real roots then these are the only solutions even if you allowed x and y to be complex numbers = x^3 3x^2y 3xy^2 y^3 = x^3 y^3 3xy(x y) Also, Read Cube of a Binomial Cube of Sum of Two Binomials Examples 1 Determine the expansion of (x 2y)^3 Solution The given expression is (x 2y)^3 We have an equation on cubes like (x y)^3 = x^3 y^3 3xy(x y) By comparing the above expression with the (x y)^3 Here, xTutor Contact tutor 7 months ago Use identity ( a b)^3 = a^3 b^3 3ab (a b ) Put a= x and b= y ( x y)^3 = x^3 y^3 3xy ( x y ) In further step 3xy can be multiplied inside the bracket The answer is 👍 Helpful



Relplot Equation Plotter




Factorise X Y 3 X 3 Y 3 3xy X Y Brainly In
Xy=7 (xy)³=7³ =343 (xy)³=(xy)(x²2xyy²) =x³2x²yxy²x²y2xy²y³ =x³y³3xy(xy) x³y³=3433xy(xy) =3433(7) =364Solution (By Examveda Team) Given, xy = 2 cubing both sides (xy) 3 = 2 3 => x 3 y 3 3xy ( xy) = 8 => x 3 y 3 3×15×2= 8 => x 3 y 3 90 = 8 => x 3 y 3 = 0 Identity (x – y) 3 = x 3 – y 3 – 3xy(x – y) (1000 – 2) 3 = 1000 3 – 2 3 – 3*1000*2(1000 – 2) = – 8 – 6000(1000 – 2) = – 8 – 100 = Factorise each of the following (i) 8a 3 b 3 12a 2 b 6ab 2 (ii) 8a 3 – b 3 – 12a 2 b 6ab 2 (iii) 27 – 125a 3 – 135a 225a 2
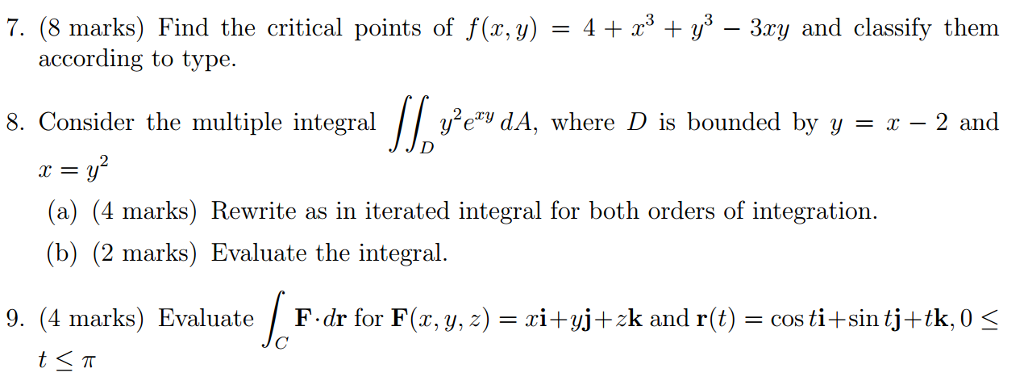



Find The Critical Points Of F X Y 4 X 3 Y 3 Chegg Com




If X 3 Y 3 1 3xy Where X Y Determine The Value Of X Y 1
2y=2 y=1 Hence, putting the value of y again in any of the above three equations will give the value of x So, taking equation x=y5 x=1–5 x=4 So the final answers are x=4 and y=1 For any clarification, please update in comments And if you liked the answer do upvote and sorry for any grammatical mistakes
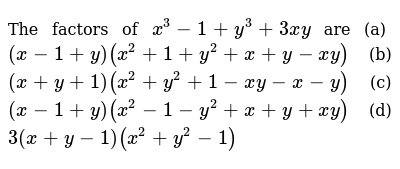



The Factors Of X 3 1 Y 3 3x Y Are A X 1 Y X 2 1 Y 2 X Y
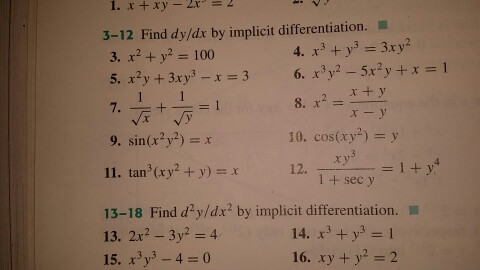



Find Dy Dx By Implicit Differentiation X 2 Y 2 Chegg Com
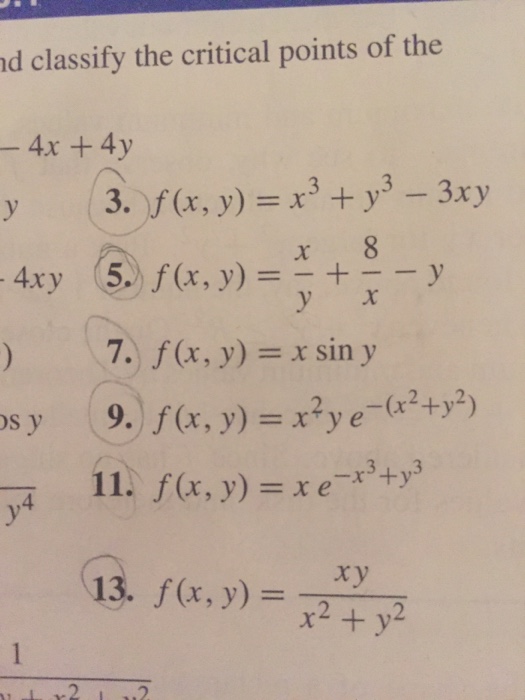



D Classify The Critical Points Of The 4x 4y Y 4m Chegg Com



How To Solve X 3 3xy 2 Dx 3x 2y Y 3 Dy Quora




Analyze The Product Of X 3 9y 3 3xy X Y
.png)



X X3 Y3 3xy X Y Maths Polynomials Meritnation Com




The Number Of Critical Points Of F X Y X 3 Chegg Com



The Two Curves X 3 3xy 2 2 0 And 3x 2y Y 3 2 0 Youtube



If X Y 2 Then What Is The Value Of X Y 6xy Quora




Transform X 3 Y 3 3xy 0 Mathematics Stack Exchange
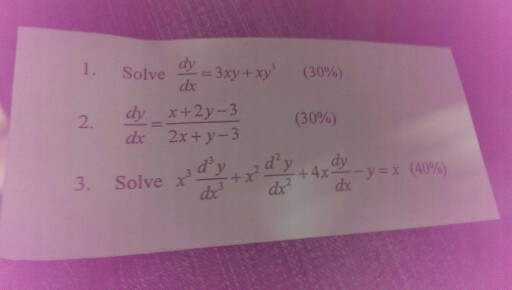



Solve Dy Dx 3xy Xy 3 30 Dy Dx X 2y 3 2x Chegg Com




Let F X Y X 3 3x 2y 3 2y 2 We Find Fx X Y 3x 2 Chegg Com




Find All Values Of X Y Where Theorem 3 1 1 The Chegg Com




Y3 3xy2 X3 3x2y Find Dy Dx Plzzzzzzzzzzz Slove Ths As Fast As Possible Maths Continuity And Differentiability Meritnation Com
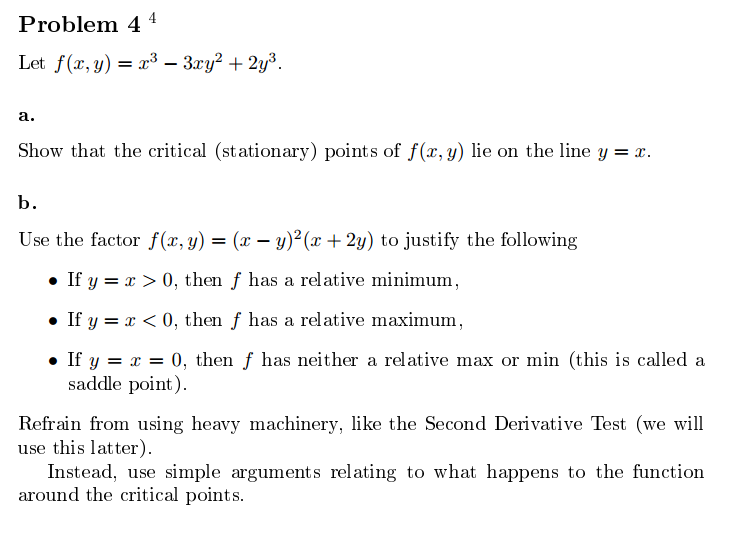



Let F X Y X 3 3xy 2 2y 3 Show That The Chegg Com




K 6y Y 1 7y 7y Jndei M 3x 4x N 6x X Je Gauthmath



1




X 3 3x 2 X 3 Factorise Novocom Top



Class 9 Chapter 2 Polynomials Cubic Identities X Y 3 X 3 Y 3 3xy X Y X Y 3 X 3 Y 3 3xy X Y Youtube



1
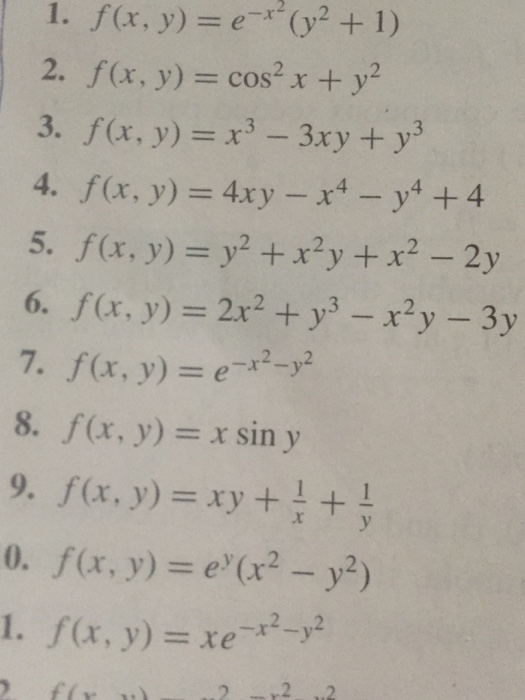



F X Y E X 2 Y 2 1 F X Y Cos 2 X Y 2 Chegg Com
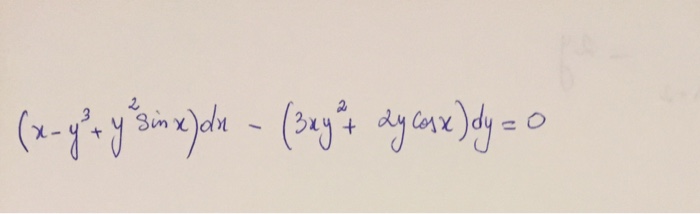



X Y 3 Y 2 Sin X Dx 3xy 2 2y Cosx Dy 0 Chegg Com



If Math X 3 Y 3 3xy 1 Math What Is The Minimum Value Of Math X 2 Y 2 Math Quora
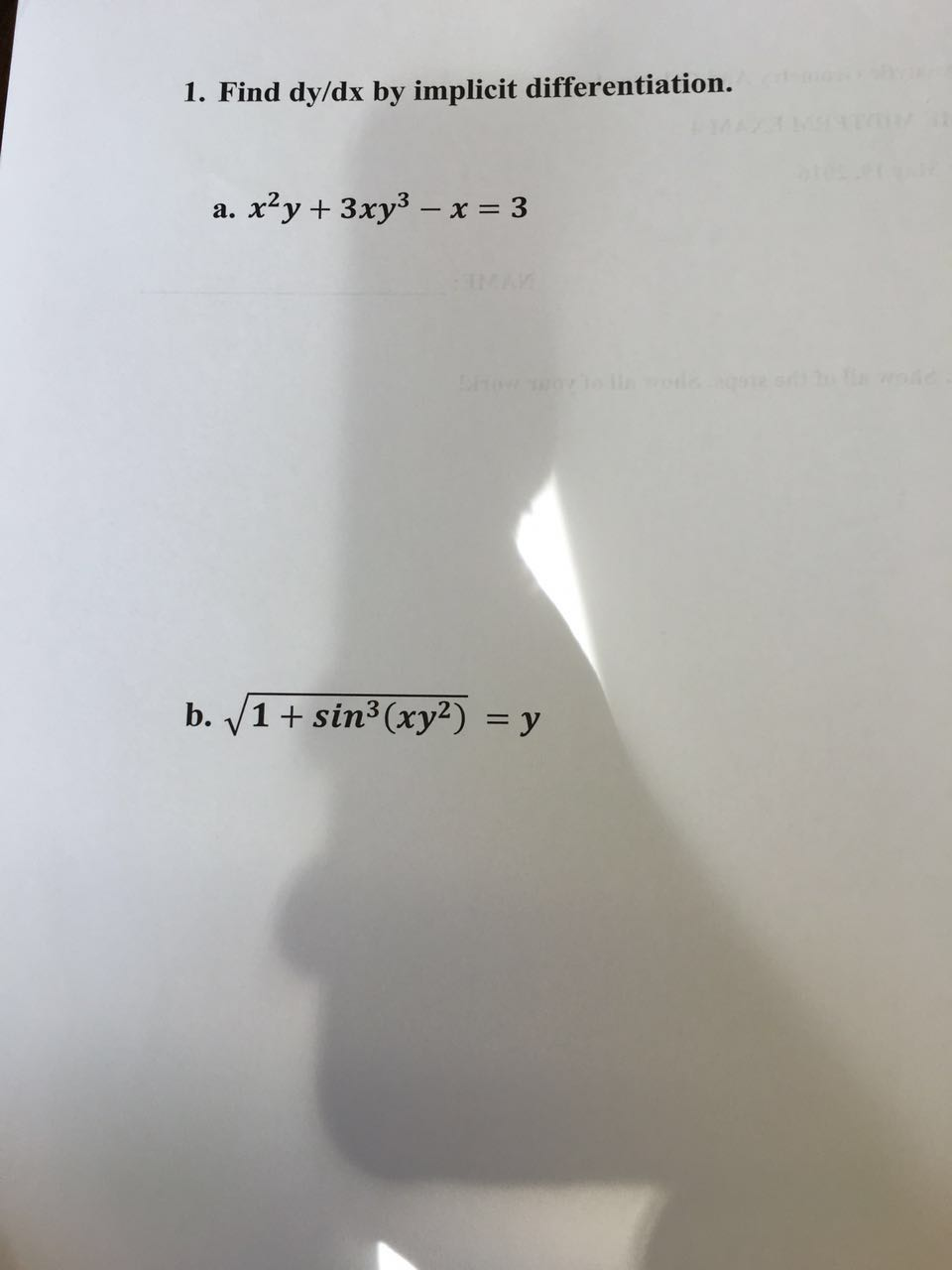



Solved Find Dy Dx By Implicit Differentiation X 2y 3xy Chegg Com




How To Solve Math X 3 Y 3 Dx 3xy 2dy 0 Quora
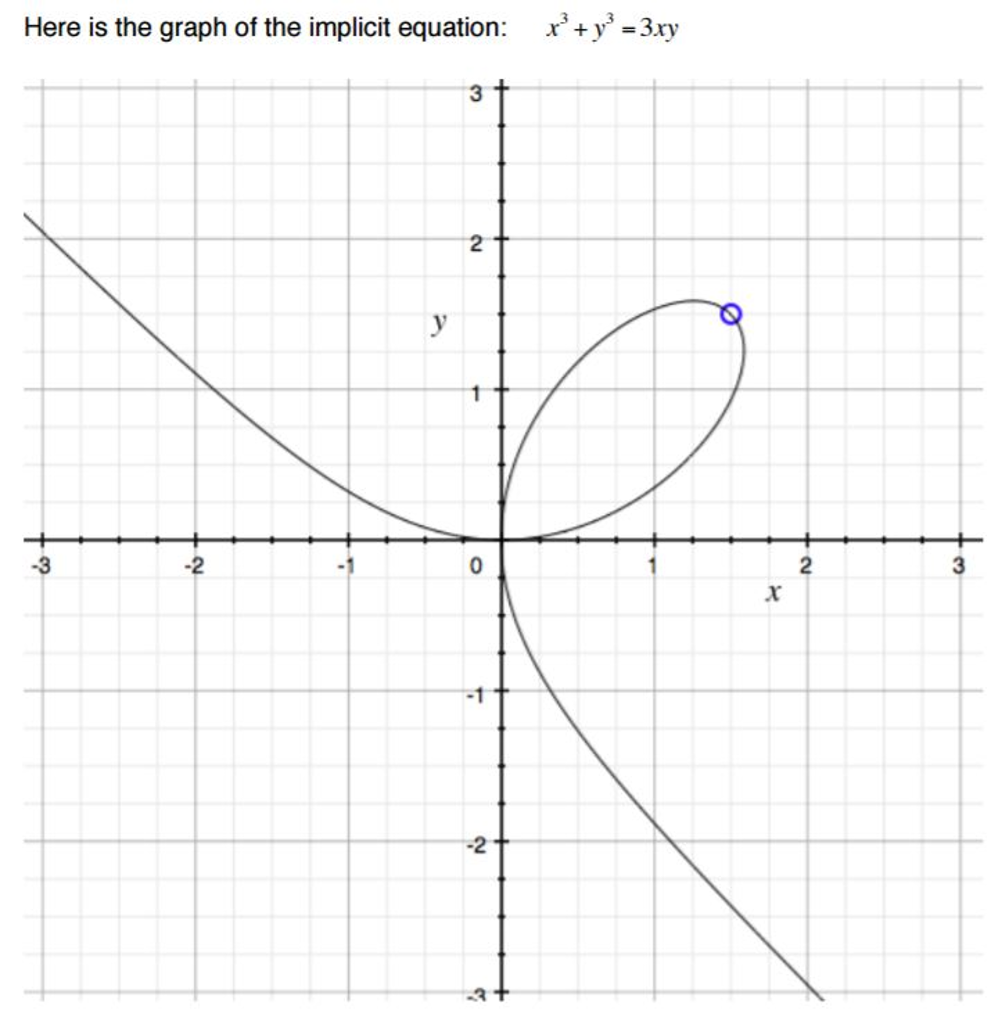



Here Is The Graph Of The Implicit Equation X 3 Y 3 Chegg Com




X 3 3x 2 X 3 Factorise Novocom Top




Find Dydx Where X 3 Y 3 3xy 7




Prove That X2 Y2 C X2 Y2 2 Is The General Solution Of The Differential Equation X3 3xy2 Dx Y3 3x2y Dy Where C Is A Parameter From Class




Factories X3 9y3 3xy X Y Brainly In
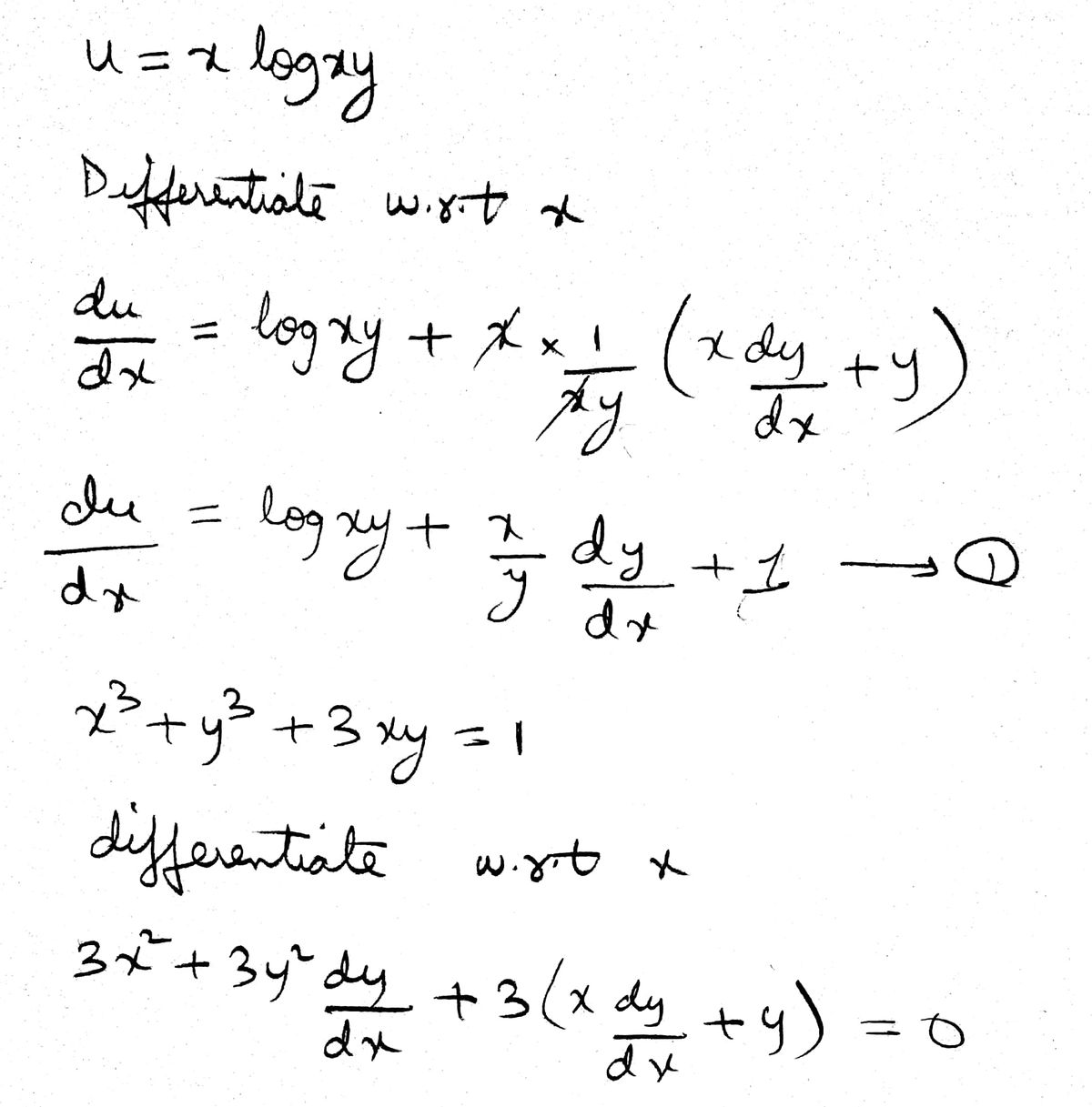



Answered If U X Log Xy Where X3 Y3 3xy 1 Find Bartleby
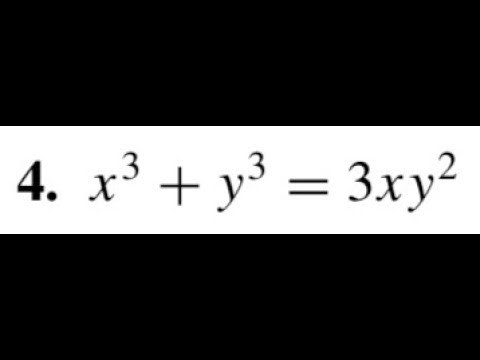



X 3 Y 3 3xy 2 Find Dy Dx Using Implicit Differentiation Youtube




If X 3 Y 3 3xy 2 3x 2y 1 0 Then At 0 1 Dy Dx Youtube



If A Line Y 3 X Cuts The Curve X 3 Y 3 3xy 5x 2 3y 2 4x 5y 1 0 At The Points A B And C




If X 3 Y 2 3xy X Y Then Log X Y 3




X X3 Y3 3xy X Y Maths Polynomials Meritnation Com




How To Factorise Using The Identity X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Xy Yz Zx Youtube




X Y 3 X3 Y3 3xy X Y Proof It Maths Polynomials Meritnation Com




How To Verify Algebric Identity X Y 3 X3 3xy X Y Y3 Maths Polynomials Meritnation Com
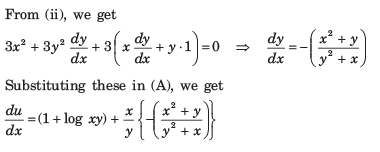



If U X Log Xy Wherex3 Y3 3xy 1 Then Du Dx Is Equal Toa B C D Correct Answer Is Option A Can You Explain This Answer Edurev Electronics And Communication Engineering Ece
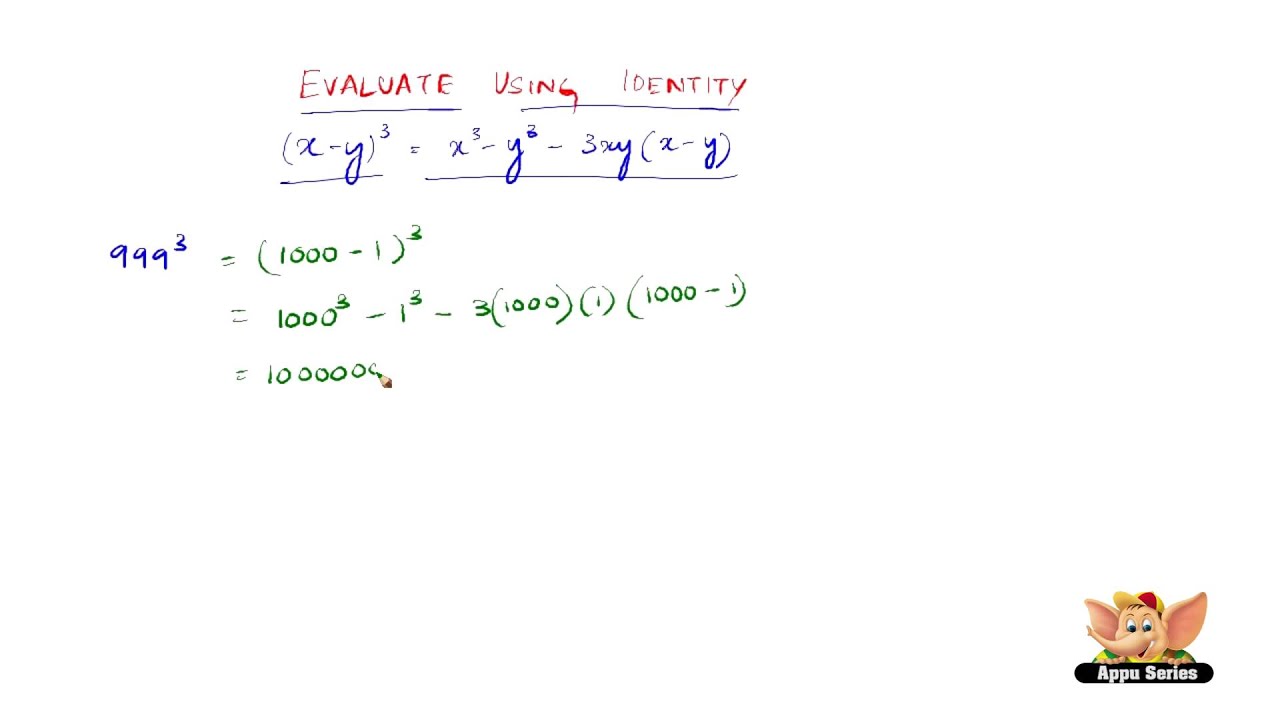



How To Evaluate Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube




Which Of The Following Is A Factor Of X Y 3 X 3 Y 3




The Factors Of X 3 1 Y 3 3x Y Are A X 1 Y X 2 1 Y 2 X Y
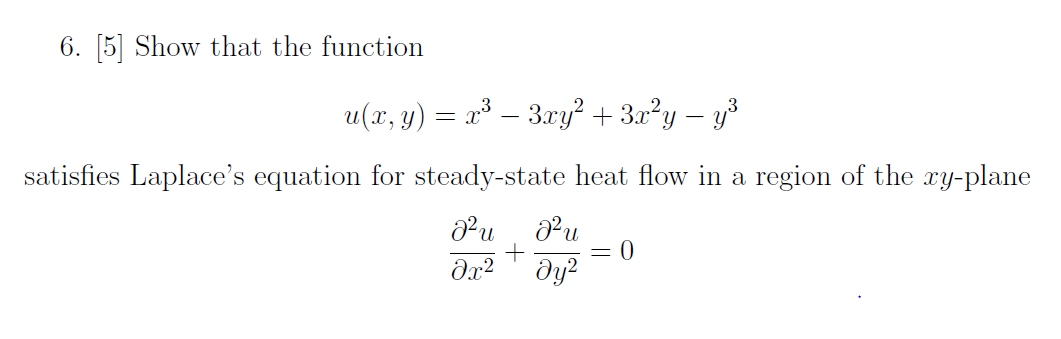



Show That The Function U X Y X 3 3xy 2 3x 2y Chegg Com



Solve X 3 3xy 2 Dx Y 3 3x 2y Dy Sarthaks Econnect Largest Online Education Community



Prove That F X Y X 3 2x 2y 3xy 2 Y 3 Is Homogeneous What Is The Degree Verify Euler S Theorem For F Sarthaks Econnect Largest Online Education Community




Factorise X X3 Y3 3xy X Y Brainly In




X 3 3xy 2 Dx Y 3 3x 2y Dy Youtube




X X3 Y3 3xy X Y Brainly In
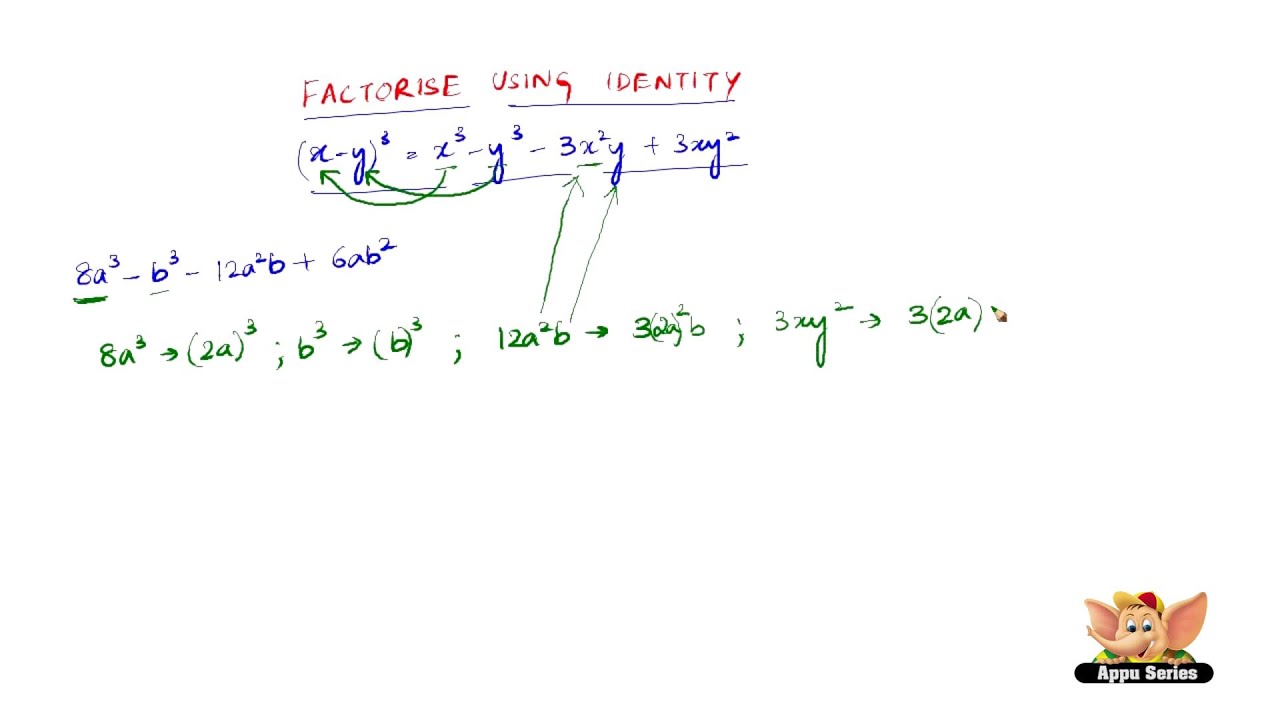



How To Factorise Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube



Factorize X X 3 Y 3 3xy X Y Sarthaks Econnect Largest Online Education Community




Implicit Differentiation Find Dy Dx If Y 2 3xy X 2 7 Chegg Com




Solve X 3 2y 3 Dx 3xy 2dy 0




Ppt Polynomials Powerpoint Presentation Free Download Id




Factorise X3 9y3 3xy X Y Brainly In
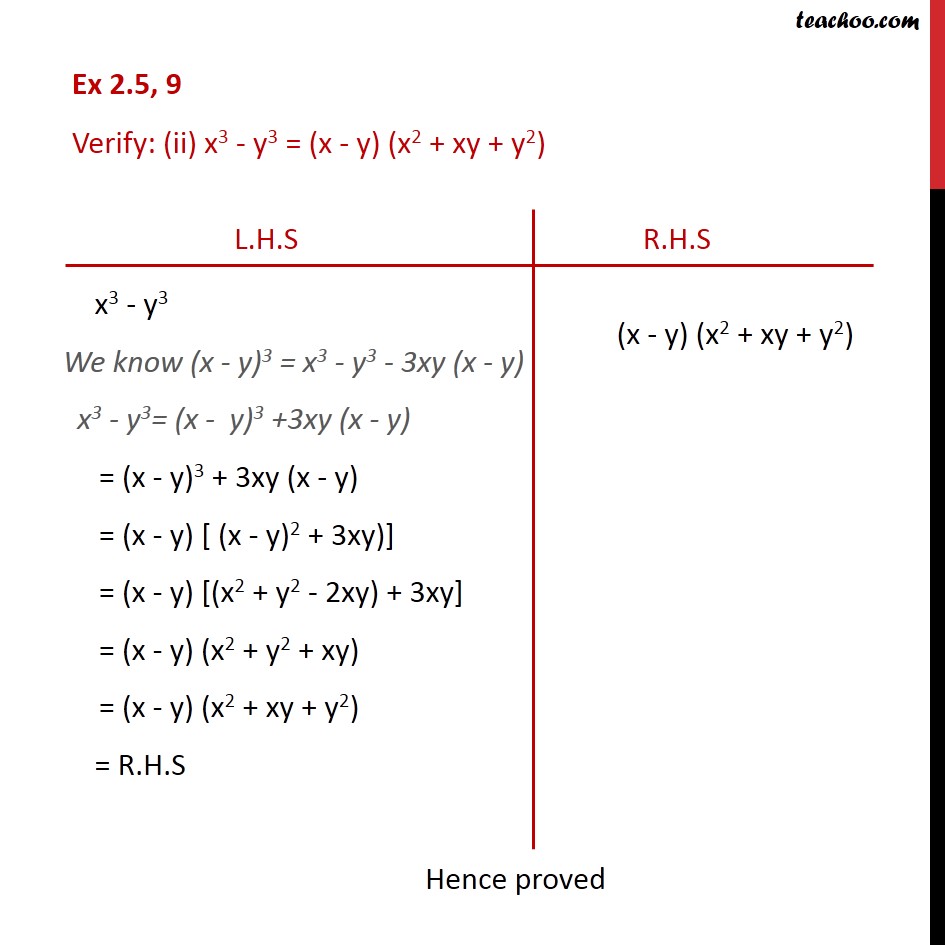



Ex 2 5 9 Verify I X3 Y3 X Y X2 Xy Y2 Ex 2 5




Find X3 Y3 3xy If X Y 1 Brainly In
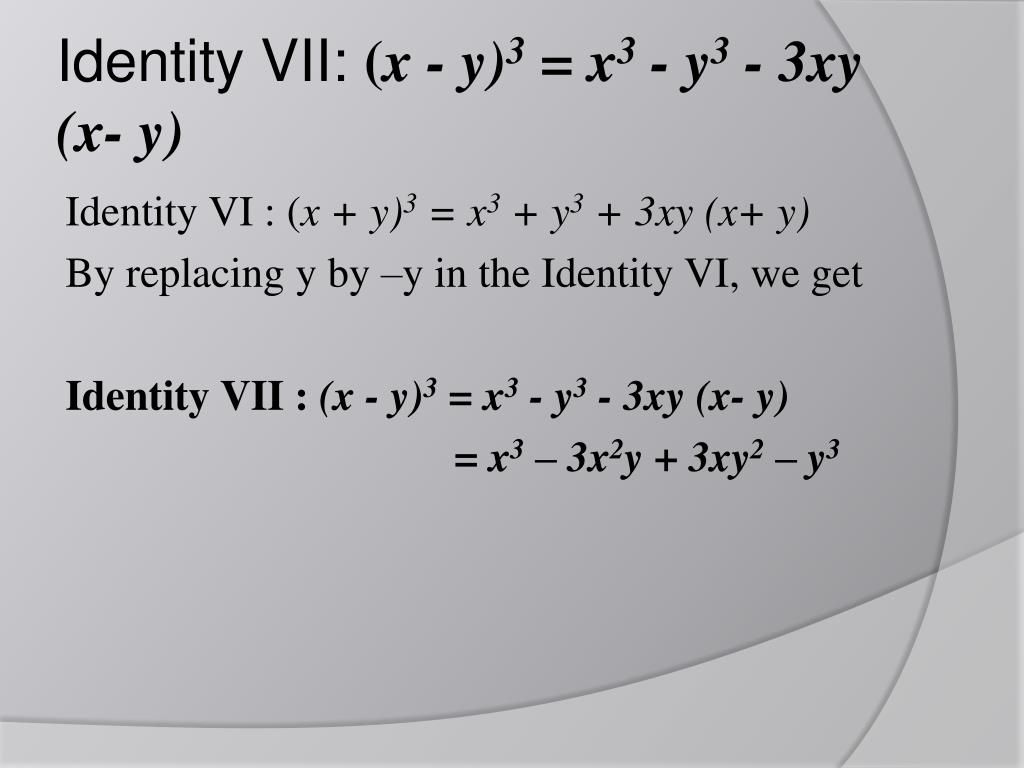



Ppt Polynomials Powerpoint Presentation Free Download Id




X 3 Y 3 Z 3 Novocom Top




X Y 3 X3 Y3 3xy X Y Brainly In




How To Know At A Glance That Math X 3 3xy 2 2y 3 Math Is Factorable Quora



Find The Extreme Values Of The Function X 3 Y 3 3xy Sarthaks Econnect Largest Online Education Community




Consider The Function F X Y X 3 Y 3 3xy Chegg Com




Factorise X3 9y3 3xy X Y Brainly In



1




3 A Classify Each Ode By Order And Linearity Y 3xy Xy 0 Homeworklib




Find Du Dx When U Xlogxy Where X 3 Y 3 3xy 1 Brainly In



How To Draw The Graph Of X 3 Y 3 3xy Quora




Find The Local Extrema Of F X Y X 3 3xy Y 3 Chegg Com
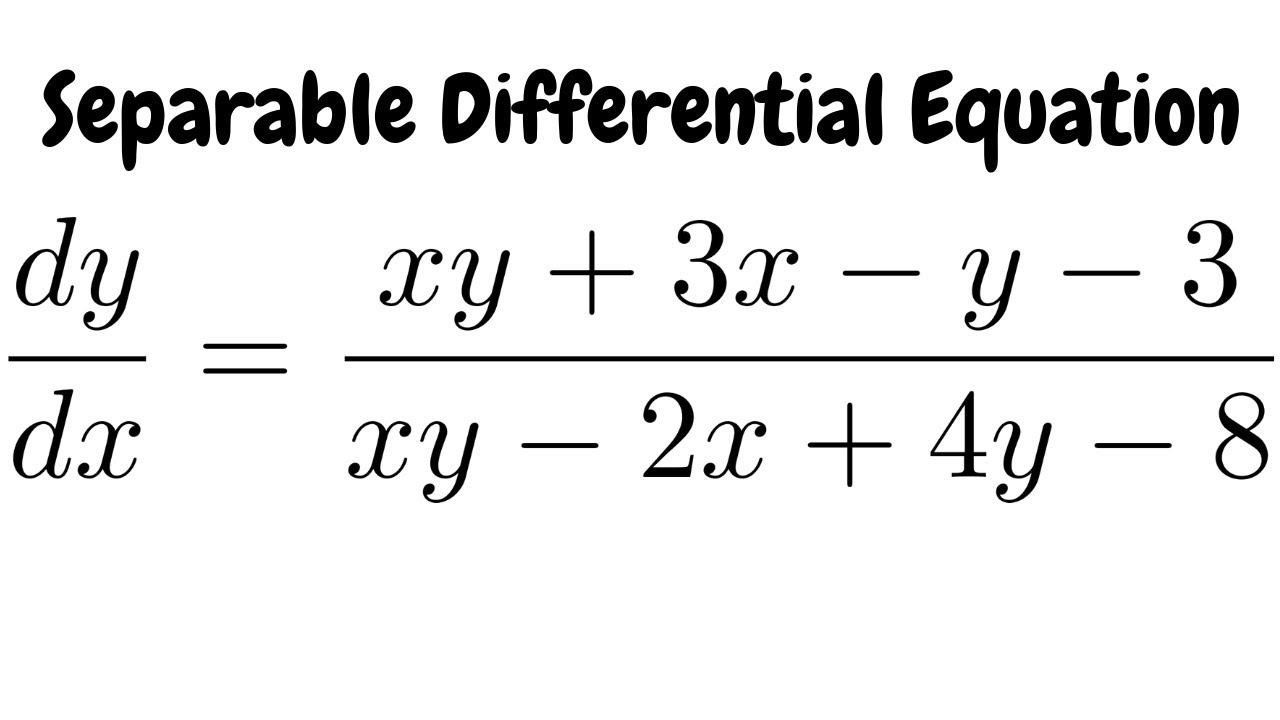



Separable Differential Equation Dy Dx Xy 3x Y 3 Xy 2x 4y 8 Youtube



If X Y 12 And Xy 27 Then Find The Value Of X3 Y3 Polynomials Maths Class 9
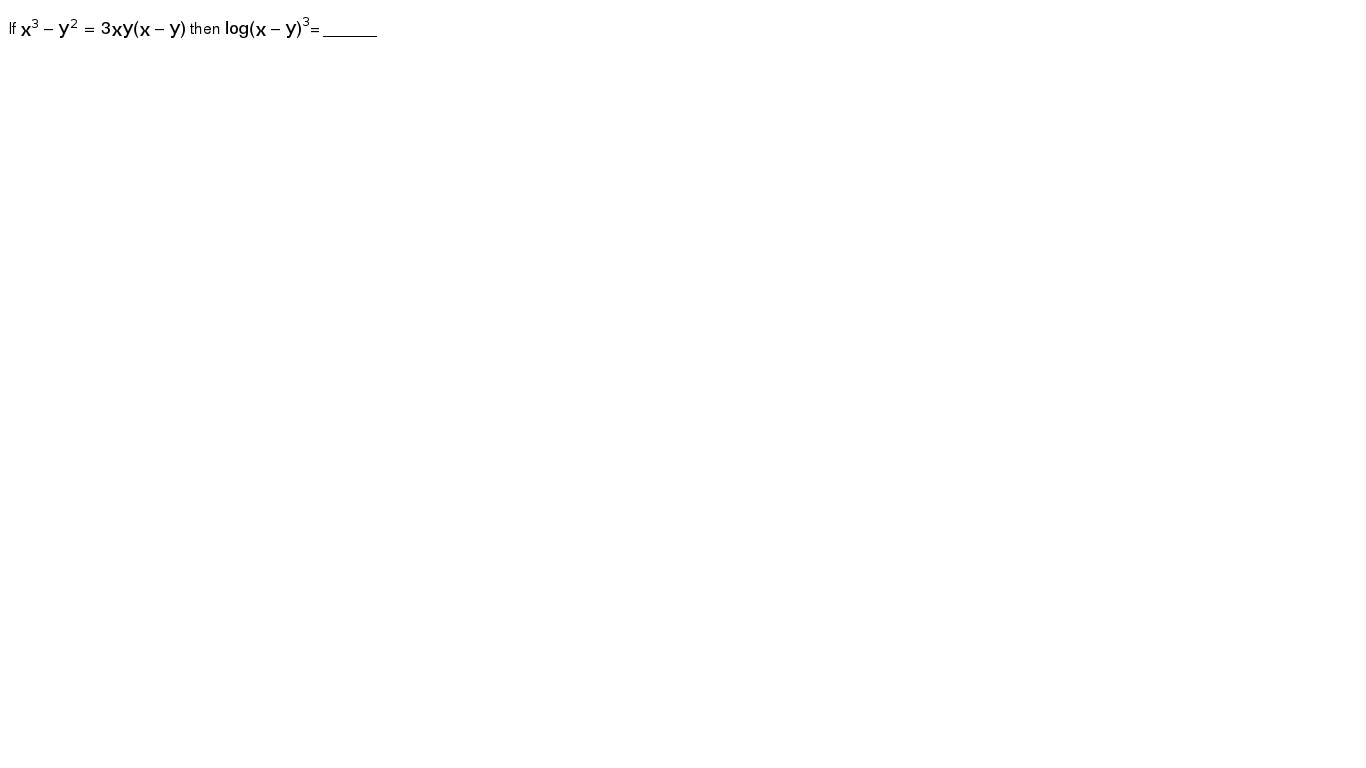



If X 3 Y 2 3xy X Y Then Log X Y 3




Solve Differential Equation X 3 3xy 2 Dx Y 3 3x 2y Dy Maths Differential Equations Meritnation Com




How To Solve Math X 3 Y 3 Dx 3xy 2dy 0 Quora


